IONISING RADIATION
Leonard I. Grossweiner, Ph.D.
Wenske Laser Center, Advocate/Ravenswood Hospital Medical Center, 4550 North Winchester Avenue, Chicago, IL 60640, U.S.A.
e-mail: jonesL@cofc.edu
1. INTRODUCTION
Ionising radiation affects all life on earth. This radiation is always present in the environment as cosmic rays from outer space, emanations from radioactive minerals, and internally from natural radioactive elements. Humans are exposed to artificial sources of ionising radiation from nuclear power plants, atomic bomb test fallout, medical x-rays, and radioactive sources used for cancer treatments. "Permissible" levels of radiation exposure are controversial, although we are better off with limits than without them. The existence of a "safe" radiation dose has been debated for decades. Experiments to test for the existence of a safe threshold dose are inconclusive. The most practical approach is to limit human exposure to ionising radiation and hope for the best. With these issues in mind, it is relevant to consider the basic nature of ionising radiation, how it interacts with matter, and the physical, chemical, and biological consequences.
Nuclear particles and electromagnetic radiation (EMR) comprise the ionising radiations. The designation "ionising" is too restrictive because the process of ionisation refers specifically to the ejection of an electron from an atom or molecule thereby creating an ion-pair. Ionising radiation may activate diverse physical and chemical effects in matter including heat generation, atomic displacements, electronic excitation of atoms and molecules, breaking of chemical bonds, and nuclear reactions. The specific effects depend on the type of radiation, the target, and the irradiation conditions. For example, natural uranium captures energetic or "fast" neutrons leading to the production of transuranic elements while
low-energy or "thermal " neutrons split uranium atoms by nuclear fission. The interactions of ionising radiation with matter always take place at the level of individual atoms. Except for nuclear reactions, the energy scale is many orders of magnitude smaller than the kinetic energy of an ionising entity. Accordingly, a single interaction can initiate a multiplicity of effects. Attempts to sort out the interaction pathways have led to much research on the radiation physics and chemistry of model systems, including pure inorganic and organic compounds, active biomolecules, cultured cells, microorganisms, plants, and experimental animals. Radiation science is important in the "real world". Radiation chemistry has led to applications in industrial processing, e.g., fabrication of plastics and radiation sterilization of food and medical supplies. Radiation damage to materials is of major interest in the nuclear power industry. Radiation biology is concerned with the damaging effects of ionising radiation on biological systems at all levels of complexity. There is a vast literature on the various aspects radiation science. The objective of this review is to provide a brief survey of the entire subject. Many of the topics are treated in general textbooks while others appear only in the scientific literature.1.1. Energetics of Ionising Radiation
The properties of elementary particles which are most relevant for their interactions with matter are the type of particle and its kinetic energy (
Table 1). The proton has a rest mass of 1.008 atomic mass units (au). One electronic charge unit (e) equals 1.602 x 10-19 coulombs (C). The electron-volt (eV) unit of energy is used extensively in radiation science: 1 eV = 1.602 x 10-19 joules (J). In thermochemical units, an energy expenditure of 1 eV per molecule provides 96.49 kJ per mole. The energy associated with EMR may be described by its wave-like properties in energy and power units or in terms of its particle-like equivalent, the photon. A useful conversion between wavelength and photon energy is E (eV) = 1239.9/l (nm), e.g., green light of 550 nm has a photon energy of 2.25 eV and a 0.1 nm x-ray has a photon energy of 12.4 keV.1.2. Radiation Dosimetry
Dosimetry is an essential part of radiation science. The roentgen (R) was the first unit of exposure dose. The roentgen is defined by the number of ions produced by x-rays and gamma-rays in a certain quantity of air under specified conditions. This unit is difficult to measure and not often used today. Fluence (F) is a unit of exposure dose defined as the number of particles or amount of energy incident per unit area. Fluence rate is the fluence per unit time. "Flux" is often used in neutron physics in lieu of fluence rate. This usage is confusing because radiant flux is a power unit in optical physics with the units of watts. The rad was the original unit of absorbed dose. One rad corresponds to the absorption of 6.242 x 1013 eV by one gram of material for any type of ionising radiation. Although the basic concept of the rad is unambiguous, it is often difficult to calculate the absorbed dose in a given system. Absorbed dose is an average quantity for a macroscopic material. The local dose in different regions of the absorber may vary owing to the production of secondary ionising particles or EMR which contribute to the dose in other regions of the material. One R of high-voltage or "hard" x-rays or gamma-rays delivers 0.87 rad to air and 0.97 rad to water. The current unit of absorbed dose is the gray (Gy); 1 Gy = 100 rad = 1 J per kg. Other units of absorbed dose have been employed to express the biological effectiveness of a given radiation. The rem (roentgen–equivalent-man) is the dose in rads multiplied by a relative biological effectiveness (RBE) factor. The RBE is taken as unity for "hard" x-rays and gamma-rays and may be much higher for other types of radiation, e.g., the RBE is about 20 for fast neutrons. An alternative unit is the sievert (Sv) which equals 100 rem, Thus, the dose in Sv and Gy are the same for RBE = 1. The maximum permissible lifetime radiation exposure is 5 mSv in the U.S. for the general population, which is equivalent to 0.5 rads of x-rays and gamma-rays. This is 0.1% of the expected lethal dose for a whole body exposure. G-value is the basic unit of radiation chemical yield. G = 1 indicates that one entity (e.g., one free radical of a given type ) is formed or destroyed for each 100 eV of energy absorbed by the medium, e.g., exposure of pure water to gamma-rays generates hydroxyl free radicals with GOH = 2.8. One G unit corresponds to a product yield of 1.0364 x 10-7 moles of the given species for each absorbed joule of radiation energy.
| Particle | Rest Mass (au) | Charge |
| Electron | 0.00055 | - e |
| Positron | 0.00055 | + e |
| Proton | 1.008 | + e |
| Neutron | 1.009 | 0 |
| Deuteron | 2.014 | + e |
| Alpha | 4.004 | + 2e |
Table 1. Rest mass and charge of some elementary particles
1.2. Natural Radioactivity
In 1896, one year after Röntgen discovered x-rays, Becquerel observed that the proximity of a photographic emulsion to uranium salts leads to blackening. He classified the emissions according to their penetrating power. Emanations stopped by a sheet of paper were termed alpha-rays (
a); those stopped by a millimeter of metal were beta-rays (b); those penetrating a centimeter of metal were gamma-rays (g). In 1909 Rutherford demonstrated that alpha-rays are energetic helium nuclei by trapping uranium emissions in a thin-walled glass vessel and exciting the optical emission spectrum of atomic helium by an electric arc. He showed also that gamma-rays can be diffracted by an ionic crystal and therefore are EMR. The unambiguous identification of beta-rays as fast electrons was finally accomplished in the 1940s by measurements of the charge-to-mass ratio. In 1903 Rutherford and Soddy demonstrated that natural radioactivity is the result of nuclear transformations. An element AXZ is characterized by its atomic number (Z) equal to the number of protons in the nucleus (and the same number of extra-nuclear electrons) and its mass number (A) equal to the total number of neutrons plus protons in the nucleus. The use of Z and the chemical symbol is redundant, although it is often convenient for displaying nuclear reactions. Nuclei having the same Z and different A are isotopes. They belong to the same chemical element and have similar properties, except for the small effects of atomic mass. Nuclei having the same A and different Z are isobars. Isobars usually have different chemical properties, although their nuclear reactions may be similar. A strong attractive force between nucleons is the glue that holds nuclei together. (Nucleons are comprised of quarks which are not relevant to the present subject.) The repulsive force between protons is a destabilizing force. Small nuclei generally have approximately equal numbers of neutrons and proton, e.g.,16O8 has 8 protons and 8 neutrons. Heavy nuclei require more neutrons for stability, e.g., 238U92 with 92 protons and 146 neutrons is radioactive.
| Isotope | Half life | Principal Radiation Emitted MeV |
| Natural isotopes | ||
| Polonium-210 | 138 days | a, 5.304 |
| Radium-226 | 1620 years | a 4.777 (94.3%) a , 4.589 (5.7%)g , 0.188 ( » 4%) |
| Radon-222 | 3.83 days | a, 5.49 |
| Artificial isotopes | ||
| Caesium-137 | 30 years | b, 1.18 (max)(8%) b , 0.52 (max)(92%)g, 0.6616 (82%) |
| Cobalt-60 | 5.27 years | b, 0.315 (max) g 1.332g , 1.173 |
| Hydrogen-3 (tritium) | 12.26 years | b, 0.0018 (max) |
| Phosphorous-32 | 14.22 days | b, 1.710 (max) |
| Strontium-90 + Yttrium-90 | 28.0 years (90Y, 64 hours) | b, 0.544 (max) b , 2,25 (max) |
| Sulphur-35 | 87.2 days | b, 0.167 (max) |
Table 2. Some radioactive isotopes used as sources. (Adapted from Spinks and R. J. Woods.)
Nuclei may be stabilized by emitting an alpha-particle which decreases A by 4 and Z by two:
A
XZ v A-4XZ-2 + 4He2Beta-ray emission which increases Z by 1 and does not affect A:
A
XZ v AXZ+1 + e-The emission of alpha-particles and beta-particles may leave the "daughter" element in an excited state. The excess energy is released by the emission of additional beta-rays and/or gamma-rays. There are three "families" of natural radioactive elements each starting with a long-lived radioactive "parent" and ending with a stable "daughter". The "primary" radioactive elements have existed for several hundred million years. They include the alpha emitters 238U, 235U, 232Th, and 147Sm and the beta emitters 40K, 187 Rb, 138 La, 115In, 176Lu, and 167Re. The "secondary" radioactive elements are decay products of the primary radioactive elements with geologically shorter lifetimes. There are three "families" of natural radioactive elements. Those elements are derived from 235U are members of the uranium or radium series; those from 238U form the actinium series; those from 232Th form the thorium series. New radioactive series are identified with transuranic elements. For example, 94Pu141 undergoes a long sequence of transformations to stable 209Bi 83. Some radioactive isotopes commonly used as sources in industry and medicine are listed in
Table 2.Most of the alpha-particles emitted by a given nucleus have approximately the same kinetic energy. In the elementary theory of alpha decay the nucleus is viewed as a potential well containing neutrons and protons. In alpha-decay two neutrons and two protons are released as a helium nucleus 4He2. This particle penetrates the wall of the nuclear potential well via the quantum-mechanical process of tunneling. Once outside the nucleus the alpha-particle gains kinetic energy owing to the repulsive force between the positively charged nucleus and the alpha-particle. Beta-rays have a wide distribution of energies (E) up to a maximum E
b (Fig. 1). Beta-emission is equivalent to the release of an electron from a nucleus accompanied by conversion of a neutron to a proton. The broad energy spectrum of beta-decay appears to violate energy conservation for those electrons having E < Eb. In 1930 Pauli postulated that the excess momentum and energy are carried away by the simultaneous emission of weightless, uncharged particles, moving with the speed of light termed neutrinos. The interactions of the neutrino are very weak and do not affect usual radiation physics and chemistry.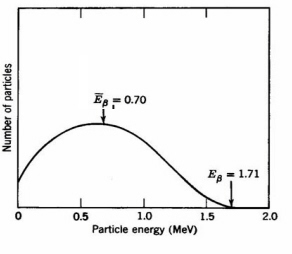
Figure 1. Energy spectrum of 32P b-particles. The average energy of 0.70 MeV is usually used for calulations of radiation damage effects (Adapted from Spinks and Woods.)
The activity of a radioactive source is the rate of nuclear decays. One curie (Ci) of radioactivity is defined as exactly 3.7 x 1010 decays per second, which approximates the activity of one gram of radium. The half-life (T) of a radioactive element is the time required for the decay of one-half of any starting number of atoms. High activity corresponds to a short half-life and vice-versa. The actual number of atoms in a given sample at any time depends on the formation and decay reactions. In "secular equilibrium" a long-lived parent generates a short-lived daughter. Since the depletion of the parent is very slow in practical times and the daughters do not accumulate, there is an almost constant ratio of daughter-to-parent atoms. An important example of secular equilibrium is the formation of gaseous radon (222Rn86) with a half-life of 3.8 days from radium (226Ra88) with a half-life of 1620 years. Radiation dosimetry for an internal source is controlled by the activity, exposure time, and the geometry. The absorbed dose rate (R) for a small source inside a large region is given by: R(Gy/sec) = 0.005927 CE, where C is the specific activity
of the source in units of mCi per gram and E is mean energy released by each decay in MeV and transferred to ionising radiation. A radon source of specific activity 1 mCi/gm in equilibrium with its daughter elements releases 0.71 Gy/sec in the form of alpha-particles and beta-particles. The absorbed dose (D) depends on the exposure time. After complete decay of a short-lived source the absorbed dose is given by: D(Gy) = 0.008551 EC0T , where C0 (mCi/gm) is the initial specific activity and T(sec) is the half-life of the dcay. The "biological half-life" of a radioactive isotope depends on both the "physical half-life" and the rate of elimination of the chemical species by an organism. A short biological or physical half-life is desirable for applications of radioisotopes in nuclear medicine. The localization of 131I in the thyroid with a half-life of 8.04 days used for radiotherapy of thyroid cancer. The 28 year half-life of 38Sr is especially harmful in radioactive fallout because this element localizes in bone.Radioactive minerals were the first sources of alpha-, beta- , and gamma-rays. Inserted radium needles are still used for cancer therapy. However, external radiation from artificial sources are more generally employed for radiotherapy. A practical source of neutrons since the 1930s consists of an intimate mixture of natural radium and beryllium. The alpha-particles emitted by radium react with the beryllium nuclei leading to emission of fast neutrons:
4
He2 + 9Be4 v 12C6 + 1n0The long half-life of radium leads to a steady beam of neutrons. Low energy or thermal neutrons are obtained by surrounding the source with water or paraffin. The maximum neutron fluence rate (or "flux") achieved by this type of source is the order of 108/cm2-s. Much higher neutron fluence rates are generated in fission reactors. A chain reaction can be established in a reactor by slowing the fast neutrons to thermal energies using water, graphite, or another moderator in order to activate further fissions. Absorption of low energy or "thermal" neutrons by the low abundance uranium isotope 235U92 (0.71%) induces splitting of the uranium nuclei into fission fragments, e.g., 93Nb and 141Pr, carrying about 170 MeV of kinetic energy, accompanied by the emission of typically two additional fast neutrons. This energy is released after about 10-12 second and is largely converted to heat within an operating reactor as used for power generation. Fast neutrons are captured by the prevalent 238U92 according to the scheme:
238
U92 + 1n0 v 239U92 v 239Np93 v 239Pu94The end product 239Pu94 can undergo fission by absorbing thermal neutrons similar to 235U92.
1.3. Artificial Sources of Ionising Radiation
The
x-ray generator invented by Roentgen in 1895 was used almost immediately for medical purposes. X-rays are short wavelength EMR, typically 0.04-0.2 D . [One Dngstrom (D ) equals 0.1 mm.] In an x-ray tube, electrons emitted by a metal cathode are accelerated by a high voltage and strike a metal anode which emits x-rays. An x-ray energy spectrum consists of several sharp spikes superimposed on a continuous background (Fig. 2) The lines are emitted when an accelerated electron ejects an inner-shell electron from a target atom followed by release of an x-ray photon when the "hole" is filled by an outer-shell electron. The continuous x-ray spectrum or bremsstrahlung ("braking radiation") is generated by the rapid deceleration of fast electrons in the vicinity of the target nuclei. The most energetic x-ray photon has an energy equal to eV0 where V0 is the accelerating voltage, e.g., the shortest wavelength x-ray generated by a 100 keV accelerating voltage is 0.12 D for any anode material. The practical energy limit for conventional x-ray tubes is about 300 keV owing to problems of materials and electrostatic breakdown. More efficient devices are available for generation of higher energy photons.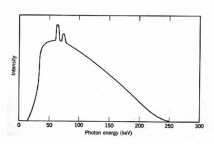
Figure 2. Typical x-ray energy spectrum for 250 KeV x-rays. The spikes are the "line spectrum" (Adapted from Spinks and Woods.)

Figure 3. Electron Van de Graaff generator. Electrons from the source (B) are sprayed on the plastic belt (C) and collected by the large metal dome (D). The accumulated negative high voltage on the dome is used to accelerate electrons from the source (E) through an evacuated tube (F). The electron beam is spread by a scanning coil (G), exit through a thin window (H) and irradiate the target (I). Adapted from O’Donnell and Sangster.)
In 1929 Van de Graaff invented an electrostatic machine for acceleration of electrons and heavy charged particles. The Van de Graaff generator is a modern version of Faraday’s famous "ice pail" experiment. A glass rod is charged by friction and touched to the inner surface of a metal pail insulated from the ground. The electric charge localizes on the outer surface of the pail where it does not impede successive transfers of charge from the rod to the inner surface of the pail. Eventually the high voltage on the pail is discharged in the form of a spark. In the Van de Graaff machine the "pail" is a large metal spherical shell charged to high voltage by collecting electrostatic charge from a rotating plastic belt located inside the shell (
Fig. 3). The accumulated voltage is used to accelerate electrons or protons generated within a long vacuum chamber located inside the shell. The maximum energy of a conventional proton Van de Graaff generator is limited by atmospheric breakdown to about 20 MeV. A tandem device doubles the useful energy by starting with negatively charged H¯ ions. The ions are first accelerated by the positively-charged shell within an external vacuum chamber, stripped of two electrons by striking a metallic foil, and then further accelerated within the shell as H+ ions.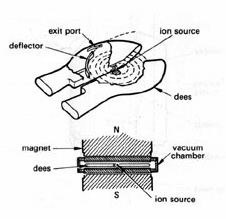
Figure 4. Cyclotron for accelerating protons. Protons from an ion source are confined to a circular orbit in a vacuum chamber by a uniform transverse magnetic field. The split metal "dees" located outside the vacuum chamber are connected to a high-voltage alternating current generator. The circulating protons receive an electrostatic "kick" each time they cross the "dees" and move to a larger orbit having the same rotational frequency. The high-energy protons are deflected out when they reach the periphery of the vacuum chamber. (Adapted from Swallow.)
Resonance accelerators employ an alternative approach to accelerate charged particles. A transverse magnetic field confines moving charged particles to a circular track. There is no energy gain, however, because the magnetic force is applied perpendicular to the direction of motion. In the cyclotron
cyclotron invented by Lawrence and Livingston in 1930, acceleration is achieved by providing an electrostatic "kick" to the circulating protons at each half revolution from a fixed-frequency high-voltage generator (Fig. 4). The device is tuned such that the frequency of the generator matches the rotational frequency of the protons. The protons move to larger circular orbits as they gain kinetic energy and eventually are ejected at the periphery of the device. This design is not practical above about 50 MeV for protons owing to the loss of tuning as the particles gain weight when they approach the speed of light. Much higher energies have been achieved with the synchrotron in which bunches of protons are confined to a fixed orbit by large ring magnet. The circulating protons are accelerated by increasing the strength of the magnetic field and the frequency of the high-voltage oscillator in synchrony to compensate for the relativistic increase of mass. Proton energies the order of 1 TeV have been attained at the Fermi National Accelerator Laboratory using a 4-mile circumference superconducting ring magnet. A cyclotron is not practical for accelerating electrons because relativistic speeds are reached at relatively low kinetic energies.The betatron is a clever device
invented by Kerst in 1950 for accelerating electrons which avoids the relativity limitation by using electric induction instead of an external electrostatic field. The basis of this effect, discovered by Michael Faraday in 1831, is that a time-varying magnetic field generates an electric field in space or in a conductor. In the betatron a transverse magnetic field confines the electrons to a circular orbit in a disk-like vacuum chamber. A tangential accelerating voltage is induced by oscillating the magnetic field at a low frequency. A small betatron operating at 300 Hz with a one meter radius generates microsecond-duration pulses of 100 MeV electrons. The linear electron accelerator or "LINAC" was invented in the 1950s to accelerate electrons. The acceleration chamber in a LINAC is an evacuated cylindrical pipe in which are located metal irises (Fig. 5). Bunches of injected electrons are accelerated along the wave guide by traveling microwaves where focussing magnets confine and direct the exit beam. A klystron is the usual source of the microwaves. This device consists is a large vacuum diode coupled to two cavity resonators. Electrons are accelerated to a potential of several hundred volts and enter a narrow gap that forms part of the first cavity resonator where they are acted upon by an applied radiofrequency field causing a bunching-up effect. Amplitude modulation of the electrons in their bunched-up state induces a high-frequency output as the electron stream passes through the gap of the second resonator which is coupled to an external transmission wave guide. A typical three meter long LINAC generates mono-energetic electron pulses of 10-6 s to 10-9 s duration in the energy range of 20-40 MeV at a repetition rate about 500 Hz. Medical LINACs generate photons by impinging the energetic electrons on a metal target.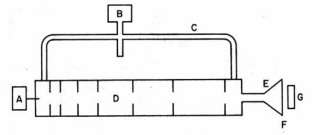
Figure 5. Linear accelerator (LINAC) for electrons. A klystron (B) connected to a waveguide (C) generates traveling microwaves in the evacuated accelerator tube (D). Electrons are injected in bunches by source (A) and are accelerated by the traveling microwaves. The pulsed electron beam is spread by a scanning coil (E), exits through a thin window (F) and irradiates the target (G). (Adapted from O’Donnell and Sangster.)
Next part